Gauss' Legacy
Gauss then went on to show that the Gaussian curvature was an intrinsic property of the surface. In other words, regardless of how you bend it (assuming that you don't cause the surface to stretch or compress), the Gaussian curvature remained the same.
This can be illustrated by looking at a simple sheet of paper. If we lay the paper down flat, it has a curvature of zero because no matter where you place the normal line on the surface, it will always point straight up (thus, the area inscribed inside the unit sphere will be a point, which has an area of 0).
If we now take this same sheet of paper and roll it into a hollow cylinder, we will see that the maximum principal curvature will now be equal to the inverse of the radius of the cylinder. Because, howver, the minimum principal curvature would remain at 0, and because the Gaussian curvature is the product of these two curvatures, it would also be equal to zero.
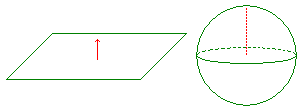
No matter where you travel on the paper surface, the normal will always point upward. Therefore, only a single point will be inscribed on the unit sphere.