The Importance of the Gaussian Curvature
Now the problem of trying to determine which side of a surface is up or down may not seem too difficult to solve when it comes to simple surfaces such as the one we have been examining thus far. But not so if we were to change our focus and look at a special geometric figure called a Mobius strip.
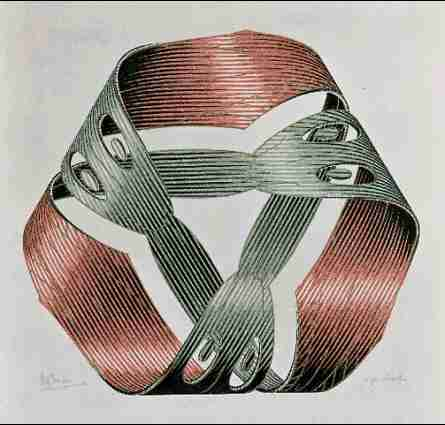
The Mobius strip is named after German mathematician August Ferdinand Mobius (1790-1868) although it was actually discovered years earlier by fellow German mathematician Johann Benedict Listing (1808-1882).
As we can see, this surface, as drawn by the famous Italian artist M.C. Escher (1898-1972) in 1961, is not quite so simple; it doesn't have a clearly defined top or bottom.
Thus, the importance of the Gaussian curvature is that it eliminated this source of ambiguity and said, in essence, it doesn't matter which side is up or which side is down.
Gauss managed to relate his definition of curvature to that of Euler's by the equation $$\kappa = \kappa_1\cdot\kappa_2$$.
This meant that regardless of the direction of the normal, both principal curvatures would change sign simultaneously (thus, both would either be positive or negative). This meant that no matter which direction the normal pointed, the curvature of the surface at that point would be invariant.