Revisiting the Definition
A Lie Group is a Differentiable Manifold which satisfies the properties of a Group, and which also has the property that its group operations are continuous.
We already know what properties make up the elements of a group (if not, see the first tutorial on Group Theory), and we just explored the definition of a differentiable manifold, but there are still a few points that need to be addressed. The first point is a minor one about the difference between a manifold and a smooth/differentiable manifold. The second point is an explanation of what it means for the operations of a group to be continuous.
Typically, a non-differentiable manifold is called a topological manifold. In a smooth manifold, the transfer functions are both continuous and differentiable (differentiability implies continuity, but not vice versa). The transfer functions of a topological manifold, however, only need to be continuous. Let's examine the function $$f\left(x\right) = \mid x \mid$$ as a brief review of continuity and differentiability.
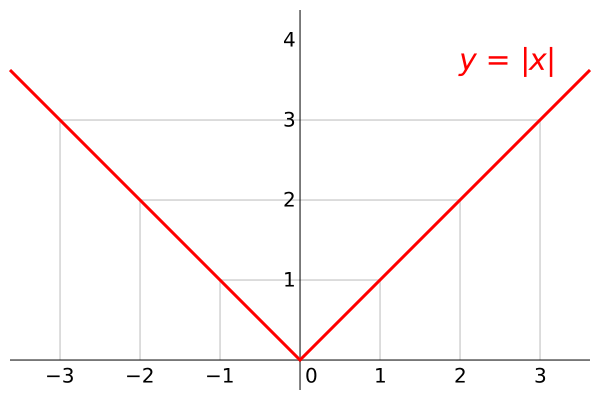
At the point x = 0, the plot is continuous but is non-differentiable.
A function f is continuous at the point a in its domain if:
- $$\lim_{x\rightarrow a}f\left(x\right)$$ exists
- $$\lim_{x\rightarrow a}f\left(x\right)=f\left(a\right)$$
By the definition, the limit as x goes to 0 of f exists. Since $$f\left(0\right)=0$$, and the limit of f as x approaches 0 from either side is 0, then the function is continuous at x = 0. Continuity is not discussed at any points not in the domain of the function being examined.
Now why is the function non-differentiable at x=0?
Let $$U$$ be an open subset of $$\mathbb{R}$$ and let $$f:U\rightarrow\mathbb{R}$$. We say that f is differentiable at $$a\in U$$ iff
exists.
But we have that $$\lim_{x\rightarrow 0^+}f^\prime\left(x\right) = 1$$ and at the same time $$\lim_{x\rightarrow 0^-}f^\prime\left(x\right) = -1$$. There is no reconciliation between the two limits, so the limit in the definition does not exist and the function is not differentiable at x=0.
Now, why should we care that the manifolds be differentiable, and not just continuous? It will become important later that we have a smooth geometry to work with in order to define smooth "flows". If the manifolds we work with have any "jagged" parts to them then it would be problematic to try and find smooth paths over them.
The second topic that needs addressing is what it means for lie groups to have the property that their group operations are continuous. An alternate way of thinking about continuity will help clarify this. If a function, f, is continuous, then no matter what point p you choose in f, you can find points as close as you want to p. So, no matter how small a distance you choose to go from p, there will always be points of f defined at that distance.
An operation is continuou if, for a point a close to c, and a point b close to d, the composition $$a\circ b$$ is close to the composition $$c \circ d$$. This property ensures that any compositions of elements of the manifold will preserve the continuous structure of the manifold.