The Surface
Now let's transfer the ideas of the tangent and normal lines from a 1-dimensional plane curve to a 3-dimensional surface. The only difference between a plane curve and a 3-dimensional surface is that instead of a single tangent line at each point, we have an infinite amount of tangent lines at every point.
This may seem to complicate things a great deal, but it actually only requires a slight modification of our current understanding.
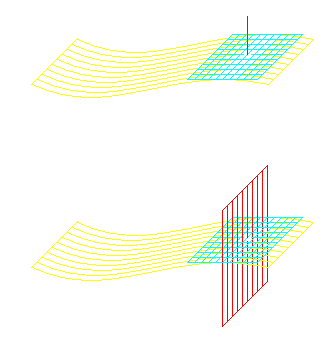
A single point on a surface may have an infinite number of tangent lines associated with it, but, as we can see in the diagram, all of these lines lie within a single plane, which we shall call the tangent plane.
While there are an infinite number of tangent lines, there is only one normal line. This is because a normal line must be perpendicular to the surface at this point, and there is only one line that can be so.
When dealing with 3-dimensional surfaces, we often talk of the nomal in terms of a normal plane instead of a single line. The concept of a normal plane comes from the idea of a plane that is normal to the tangent plane rather than the tangent line. Thus, the normal surface can have an infinite width (though it is geometrically accurate only at the vertical extending from the point of interest).