Surfaces of Constant Curvature
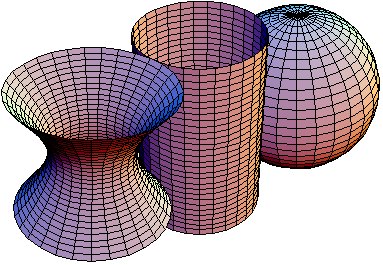
The figure on the right shows three surfaces of constant Gaussian curvature. The hyperboloid on the left has constant negative curvature, the cylinder in the middle (which can be thought of as a plane rolled so that the ends meet) has constant zero curvature, and the sphere on the right has constant positive curvature. There are two important results about such surfaces.
The first theorem, attributed to Ferdinand Minding, states that all surfaces with the same constant curvature are locally isometric. That is to say that such surfaces can be thought of as the same in some sense due to a 1-1 and onto mapping. A byproduct of this theorem is the notion that we have been dealing with of rotating the plane into a cylinder. Minding's theorem has the consequence that any surface whose curvature is zero (such as the cylinder) can be made by bending a plane region; he called these surfaces developable surfaces.
The second theorem, called Liebmann's theorem, states that spheres are the only closed surfaces of constant positive curvature. Every other surface of constant positive curvature will be open, i.e. will have a definite boundary. While less far-reaching than Minding's theorem, this denotes a special intrinsic property that spheres have.