Euler and the Principal Curvature
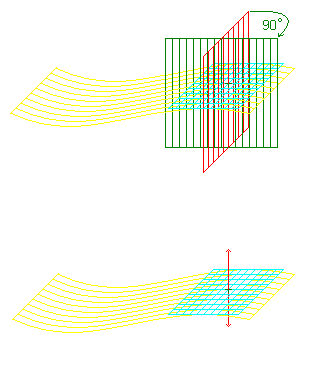
Euler discovered that the curvature of all of the curves that travelled through this point of interest could be derived using only three values: the maximum curvature, the minimum curvature, and the angle between the normal sections that these correspond to.
He later found that these normal sections were always orthogonal to one another, meaning that the angle between them was always 90 degrees!
The formula that Euler proposed for calculating the curvature at a point was:
The importance of this formula, in case it is not quite clear yet, is that the curvature at a point on a 3D surface could now be quantified using only two other values.
There remained, however, a small problem in actually attempting to measure curvature using this method: at any given point of reference, the normal line would have two directions (above and below the surface). Thus, the curvature calculated using one line would be the negative of that using the other.