The Inverse
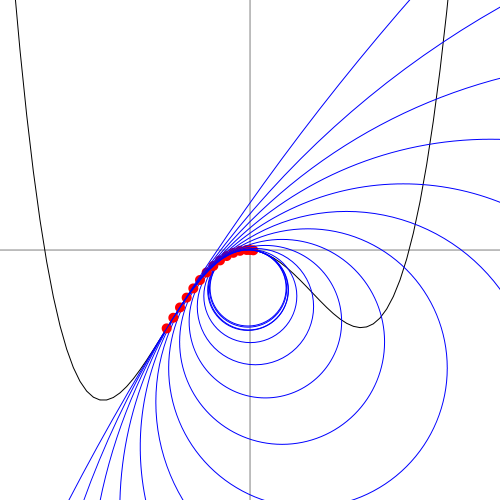
If we used the radii alone to measure the curvature of a curve, however, we would find that where the curve was straight, the radius would be infinite (a circle would have to be infinitely large in order to be tangential to a straight line). Because we know that the curvature of a straight line has to be zero, we take the inverse of this radius to be the actual curvature.
As we can see in this image using our osculating circle example, as our point of interest moves along the curve from a peak to a straighter segment, we see that the osculating circle grows larger and larger until resembling a straight line.